Which is the hardest mathematical question in the world, or math problem?
The Riemann Hypothesis is a mathematical conjecture proposed by the German mathematician Bernhard Riemann in 1859 that has puzzled mathematicians for over 150 years. It states that every nontrivial zero of the Riemann zeta function has a real part of ½.
It’s difficult to determine the “hardest” mathematical question in the world because there are so many complex and unsolved problems. However, some of the most famous unsolved problems in mathematics include the following:
1. The Riemann Hypothesis: This is one of the most famous unsolved problems in mathematics and seeks to determine the distribution of prime numbers.
2. Navier-Stokes Equations: These equations describe the motion of fluids but are extremely difficult to solve for complex systems.
3. Hodge Conjecture: This is a problem in algebraic geometry that deals with the properties of complex geometric shapes.
4. P vs. NP: This is a question about the fundamental nature of computation that asks whether problems that are easy to verify (NP) are also easy to solve (P).
5. Birch and Swinnerton-Dyer Conjecture: This conjecture has to do with the properties of elliptic curves and their associated L-functions.
These problems are difficult or impossible to solve using our current understanding of mathematics and continue to challenge some of the best mathematicians in the world.
The Sofa Problem
Say you’re moving into a new house. You’ve just finished u-hauling your furniture over and are in the process of setting up your sofa in the living room.
There’s just one problem. The hall to the living room has a 90° turn to it. No matter how hard you push, you can’t get your sofa past it. It’s stuck.

But say you really want a big sofa. What’s the largest sofa you can fit past the corner?
The answer is, no joke, the sofa is constant. It’s seriously called that in formal mathematics. And what, you ask, is the sofa constant?
We have no idea.
We’ve got some lower and upper bounds, but the specific maximum of the area that can be bent around a 90-degree turn is an open problem in mathematics
As it turns out, the question of “how can an arbitrary shape of arbitrary size be bent around a corner” is a remarkably difficult one.
Like moving wasn’t enough of a pain already.
Who is Norma in Suits Why isn’t she shown?
Which is the hardest mathematical question in the world, or math problem?
Square peg problem: ~ Can you find four points in any closed loop that form a square?
Okay, so we literally have no idea how hard unsolved problems are to solve, so this question is slightly inaccurate, but I honestly cannot imagine a simpler problem that is unsolved. You only need to know what a square and a squiggly line are to understand this. No addition no numbers, etc.
While the problem was only formalised in 1911, it has been around as long as we can tell, as its incredibly basic.
A few summers ago, we showed we could draw a rectangle in most loops; this summer, we made it to all smooth loops and all rectangles! We did this by mapping aspects into a topological shape. It turned out weirdly that the reason a rectangle works is because a mobius strip intersects itself with only one side.
I mention this problem because more progress has been made in the last few months. For more info on what we have been able to do, check out Wikipedia, which lists exactly what steps were taken and what is left!
Inscribed square problem – Wikipedia
Yet we cannot use the same method for squares. so it remains a mystery!
Edit:
I just realised I haven’t made it very clear that I am referring to all loops, including ones with corners. Loops without corners are called smooth, which is what is shown in the 3B1B video in the comments (thanks for that) Unfortunately, loops with corners, especially loops with only corners (like the Koch snowflake), are not that simple to solve!
More Edits:
It turns out that the Koch Snowflake (which I gave as an example above) has actually been solved, but other similar types of shapes have not.
And also, just to clarify for the second time, by “all” rectangles, I mean any rectangle you can draw, so in technical language, all aspect ratios.
Basically, what’s left is: can we make a square in pointy loops?
What is the hardest math problem ever?
Back in the ‘70s and before, the Mathematics Department of the University of Moscow, the Soviet Union’s most prestigious math school, was actively engaged in discrimination against bright Jewish students to keep them out of the program. They did this in quite an insidious way. In place of the standard entrance exam, they gave these “undesirable” applicants a test from a set of special problems called “coffins,” which had three very interesting (when taken together) properties:
- They could be very simply stated in terms of only elementary concepts (i.e., what math one would normally learn in secondary school).
- They had short, simple solutions that also involved only elementary concepts. That way, were someone to complain about the difficulty of the problems and raise the issue of discrimination, the examiners could show them the very simple solution as contradictory evidence.
- The solution involved an ingenious leap of intuition or clever trick that would be unlikely to be discovered in the limited time given.
The unwanted applicants would be presented with problem after problem from this collection until they could not solve one, at which point they would be rejected.
Because such problems are not that easy to create, the ones they did manage to collect were kept a very close secret for some time. However, we now have a fairly representative collection of these problems from those who came in contact with them, along with some modern additions, listed here: Main list of math “coffin” problems
Which is the hardest mathematical question in the world, or math problem?
Personally speaking, my favorite one is this Navier-Stokes equation (which describes the motion of fluid):
Just notice how many terms are in this beautiful equation. Because the NS equation is so complex, very few analytical solutions were found, and in most cases, we rely on numerical computation (aka computational fluid dynamics). However, even CFD needs to rely on some empirical laws to simplify the calculations instead of solving the NS equation from scratch (aka direct numerical simulation).
Another is this Einstein Field Equation, which governs the motion of gravity:
Although EFE seems to be much “cleaner” than the NS Equation, it’s just an illusion. Each term in the EFE is a second-order tensor, which is a 4×4 matrix made up of 10 independent components, as the figure below shows:
Unlike the NS equation, we did find some useful analytical solutions to the EFE. Examples include Schwarzschild metric (which describes a stationary, neutral black hole), Reissner-Nordström metric (a stationary, charged black hole), Kerr metric (a rotating, neutral black hole), and Kerr-Newman metric (a rotating, charged black hole). Since black holes are only described by their mass, charges, and angular momentum, all of them can be perfectly described by the above solutions. Another well-known solution is the Friedmann-Lemaître-Robertson–Walker metric, which describes our expanding universe.
If these two equations are not mind-boggling to you, what about combining them together? That’s exactly the case of a binary neutron star merger, where gravity and fluid dynamics are tightly coupled with each other. So you can understand why binary neutron star mergers are considered the “holy grail” of numerical simulation.
What is the hardest type of math?
When I first saw ito-calculus, I was amazed. It seemed so complicated! I tried reading books about stochastic integration but barely understood one out of five sentences. I kept at it, watched videos, read papers, and most importantly, I used it. Now, I can read papers involving stochastic integration and understand them just fine.
The point of this story is to illustrate my answer: The hardest kind of math is the kind you’ve never seen before.
I would say that there is a level in mathematics called the “Genius-Level Gap,” which separates already extremely difficult math from genius-level math.
After the gap, there are some concepts that I would really have difficulty grasping in an entire lifetime, and only a few people are able to deal with them.
I’m talking about stuff like homological mirror symmetry, complex Kleinian groups, perfectoid spaces, Fermat’s last theorem, the Poincaré conjecture, and so on.
The proofs behind them are the results of years and years of research involving the best minds on earth.
Maxim Kontsevich is the man behind the homological mirror symmetry conjecture.
Which is the hardest mathematical question in the world?
There’s something both thrilling and maddening about the idea of an unsolvable problem. It’s like that last boss in a video game that you just can’t seem to conquer, no matter how many combo moves you’ve masterfully executed. In mathematics, this concept takes a far more cerebral turn, with problems that have stumped minds far greater than most of us can even comprehend.
But what is the hardest mathematical question in the world? What’s that Everest of numbers and logic that mathematicians look up at in a mix of awe and frustration? Well, one could argue it’s one of the problems we’ve yet to solve: The Millennium Prize Problems.
Back in 2000, the Clay Mathematics Institute laid out seven such brutal brain busters. They called them the “Millennium Prize Problems”, and for good reason. These weren’t your average algebraic annoyances; these were Mount Olympus-high mathematical conundrums, each carrying the hefty gold medal of a $1 million prize for whoever could solve them.
To date, only one of the problems, the Poincaré Conjecture, has been definitively solved. That leaves six problems still shrouded in mystery, intellectual gloom, and the potent allure of that seven-figure carrot dangling before the world’s most brilliant minds.
In the rogue’s gallery of these problems, The Riemann Hypothesis stands out; it’s essentially the Joker of the pack. Posed by Bernhard Riemann in 1859, it’s concerned with the distribution of the zeros of the Riemann zeta function and is fundamentally connected to the distribution of the prime numbers. Despite the tireless efforts of some of the sharpest minds over more than a century, this beast remains untamed. Its solution (or proof of its unsolvability) is expected to send a shockwave through the world of mathematics, impacting everything from number theory to the security of internet encryption.
Another notorious entry is the Navier-Stokes Existence and Smoothness problem. It’s like an arcane incantation from the realm of partial differential equations, governing the motion of fluid substances. At its core, it’s a challenge to mathematically prove that, under certain conditions, the equations always have solutions, and those solutions are smooth and consistent. Sounds innocuous, but this is mathematical rocket fuel, key to understanding the flow of oceans, weather patterns, and even the blood coursing through your veins.
Attempting these problems is akin to picking a fight with the gods of mathematics. They are not just tough nuts to crack; they are veritable monoliths of cerebral fortitude, challenging our very understanding of mathematics. Whoever cracks any of these cases won’t just be known as a great mathematician; they’ll be etched in history as intellectual legends.
The truth is, these problems are about more than just the challenge or the money; they represent the frontier of human knowledge. They’re a testament to how far we’ve come and a humbling reminder of how much further we have to go.
Math, it turns out, is the ultimate endurance sport. And the Millennium Prize Problems are its Olympic Games, complete with the thrill of victory and the agony of a million dollars not quite within reach. Yet.
So, to any math enthusiasts out there feeling plucky, or to those who just want to make history — why not give it a shot? After all, with problems like these, the journey is just as rewarding as the destination. Well, almost – the destination here comes with a $1 million check.
What is the single hardest math problem you ever attempted to solve?
1970 or 71: I was in my advisor’s office for our weekly meeting on my progress in learning bordism/cobordism theory.
He turned in his chair and pulled off a shelf one of those thick yellow Springer paperbacks containing proceedings of a recent conference. Flipped to a page somewhere in the middle and pointed to a footnote.
The footnote said that “Milnor has recently proved …” the result that any graded module over the Lazard ring could be realized as the complex bordism module of a CW-spectrum, and the universal map from the Lazard ring could be realized as a map of the spectra. (By implication, Milnor had not yet published the proof.) My advisor said, can you prove this? Come back next week.
It was a test of my developing knowledge and skills in the subject. It was the sort of thing that everyone had figured ought to be true, but no one had got around to actually proving it because there were always bigger, more interesting fish to fry. Apparently Milnor had finally taken the trouble to work out the proof and my advisor was asking me to try to do the same.
I went away had one of the most exhilarating weeks of my life. Very little sleep. Saw right away that I would need to use Postnikov towers to get the proof. Intense periods of time with my Spanier making sure I was using the Postnikov techniques correctly. One way or another the details required almost every bit of algebraic topology I had learned. And in the end it all worked out. I could write down the whole proof.
At our next meeting, exhausted but excited, I bounded into my advisor’s office saying “I proved it!”
He looked puzzled. “Proved what?”
I told him “That any graded module over the Lazard ring could be realized as the complex bordism module of a CW-spectrum, and the universal map from the Lazard ring could be realized as a map of the spectra.”
He still looked puzzled. Then turned around in his chair, pulled the same yellow Springer conference proceeding off the same shelf, turned to the same footnote, and said “Milnor already proved that.” He forgot completely about having challenged me the week before to prove it. I was so confused that I didn’t even tell him that he had forgotten. I just mumbled something and went away.
Which is the hardest mathematical question in the world?
Determining the hardest mathematical question in the world is subjective and can vary depending on individual perspectives and areas of expertise. However, there are several well-known open problems in mathematics that are considered exceptionally challenging and have stumped mathematicians for many years. Here are a few examples:
1. The Riemann Hypothesis: Proposed by Bernhard Riemann in 1859, the Riemann Hypothesis is one of the most famous unsolved problems in mathematics. It deals with the distribution of prime numbers and states that all non-trivial zeros of the Riemann zeta function lie on a specific line in the complex plane. The Riemann Hypothesis has far-reaching implications and has resisted proof or disproof for over a century.
2. The Birch and Swinnerton-Dyer Conjecture: This conjecture is related to elliptic curves, which are fundamental objects in number theory. The conjecture suggests a deep connection between the arithmetic properties of elliptic curves and the behavior of their associated L-functions. It remains unsolved, and proving it would have significant implications for the study of elliptic curves and Diophantine equations.
3. The P versus NP Problem: This problem belongs to the field of computational complexity theory and asks whether every problem for which a solution can be verified efficiently (in polynomial time) can also be solved efficiently (also in polynomial time). The question essentially asks if P (problems solvable in polynomial time) is the same as NP (problems verifiable in polynomial time). Resolving this problem would have profound implications for computer science and cryptography.
4. The Navier-Stokes Existence and Smoothness Problem: This problem is concerned with the behavior of fluid flow and asks whether solutions to the Navier-Stokes equations exist for all possible initial conditions and whether these solutions remain smooth (without any discontinuities) for all time. Despite progress in understanding the equations, proving the existence and smoothness of solutions in the most general case remains an open question.
These are just a few examples of challenging unsolved problems in mathematics. It’s important to note that the notion of “hardness” can vary, and there may be other problems that some mathematicians consider the hardest. The pursuit of solving these problems drives mathematical research and fuels ongoing exploration and innovation in the field.
What’s the hardest math problem that a person has solved?
There are a number of them, since maths are like Legos and theorems build upon each others. But, Gödel’s theorem is quite an impressive one. While Hilbert had that brilliant idea to formulate an impenetrable conceptual framework that would automate the resolution of all maths problems, Gödel shattered his dream by proving it was impossible.
Yes, Gödel was Luke Skywalker derailing the grand vision of Darth Vader building a death star of mathematics. 😁
On the other hand, Gödel was a logician, so we could also cite the Wile/Fermat’s theorem as some of the most difficult undertaking in maths.
Why this one, since there are many other difficult theorems?
Maybe, because a lot of the hard theorems were hard because everybody was lacking a good idea on how to solve them, but once they had that flash of intuition, they knew they could do it. Solving Fermat’s problem was a step further, since, even with a good idea, it could not be solved, at all, with regular maths.
They had to use another kind of maths to try to solve it and Wiles spent almost a decade of his best years working on this obscure topic everybody had deemed unsolvable. Few people have the skills and desire to work on those problems but even fewer will bet their entire academic career on a problem so hard the chances of success were almost non existant.
Yet, he made it, against all odds.
Many difficult math problems have been solved throughout history, but one of the most famous and challenging problems is considered to be Fermat’s Last Theorem. This theorem was first proposed by the French mathematician Pierre de Fermat in the 17th century. It states that no positive integers satisfy the equation x^n + y^n = z^n for any value of n greater than 2. Fermat claimed to have proof for this theorem, but he did not write it down, and it remained unsolved for more than 350 years.
In 1993, Andrew Wiles, a British mathematician, announced that he had finally found proof for Fermat’s Last Theorem, after working on the problem for over seven years. Wiles’ evidence was based on a combination of techniques from algebraic geometry and number theory, and it was considered to be one of the greatest achievements in mathematics of the 20th century.
Other examples of complex problems that were solved by mathematicians are the Poincaré conjecture, Taniyama-Shimura conjecture, and the Hodge conjecture. These problems were solved by Grigori Perelman, Andrew Wiles, and Richard Taylor respectively, with the first two being awarded the Fields Medal and the last one being awarded the Abel Prize.
It’s important to note that mathematics is a constantly evolving field, and new problems are being discovered and solved all the time, so it’s hard to determine the hardest math problem that a person has solved.
What is the most difficult math question you know the answer to?
I took a course in Differential Equations my senior year in college. I needed an elective. It was the only math course open to me I had not taken. I took it on a whim because I never expected to use it in my chosen career.
6 years later I was a process engineer at a paper mill. My technical director assigned me to oversee the biocide system. Paper mills add small amounts of additives (mold and bacteria killers) to the paper machine system to keep bacterial or mold deposits from plugging anything up. We were spending a lot of money on these specialty chemicals (high cost) and setting our dosage based in the recommendations of the chemical salesman.
His recommendations were based on a laboratory study made some years earlier to determine the concentrations of certain chemicals to kill bacteria and mold The laboratory that did the study was a university lab. The salesman was guessing at the feed rate we should maintain in our machine system.
A paper machine can be described as a high volume loop of water that circulates through a large pump. Fiber is added. The water containing about 0.5% fiber goes to the paper machine where filtration is used to form a sheet of wet paper at about 13% solids (later dried). The rest of the water goes into a system of treatments and storage tanks I will call units that eventually feet the large pump.
The biocide is pumped for a few minutes each 12 hours into one of the units, and the carryover was expected to treat other units. there were 16 units within the loop of our paper machine. I wrote differential equations for the biocide concentrations in each unit. Then I had a system of 16 simultaneous differential equations with 16 variables to solve.
Taking time out for other projects under my responsibility, it took me a month to write the equations and solve the system of equations. This gave me the approximate concentrations of biocide in each unit. All were well above the recommendation of the laboratory study. We reduced biocide consumption. I saved the company a lot of money.
I was never formally taught how to solve systems of differential equations, but I learned in differential equations class how to set up and solve a differential equation, and I applied the technique from algebra for solving a system of simultaneous equations.
I have worked with many good salesmen who are informed and trustworthy, but this taught me never to trust information from a salesman without some kind of verification.
What is a math question that looks easy but is difficult?
I think I’ll go with the controversial Question 6 from the Math Olympics of 1988. This problem has received somewhat of a legendary status since its conception.
The problem is as follows:
Okay, it doesn’t look that easy, but when you put it next to problems like these:
It looks like something out of an Introduction to Algebra first set problem. But don’t let its looks fool you. Terence Tao, regarded as one of the smartest people alive, and a Fields Medal winner, scored 1 out of 7 points on the problem.
When I myself stumbled across the problem, I thought somebody was trolling the world. It looks like basic algebra. Then I tried solving it and let me tell you, it wasn’t fun.
And I wasn’t the only who thought it was a joke. When the problem was submitted to the committee responsible for putting together the Olympics exams, they were going to write it off before even solving it. Then they actually tried solving it. Let me remind you, these guys are hardcore, seasoned mathematicians. As you probably guessed by that foreshadowing, nobody managed to solve it.
So what is the solution?
Well, it’s too long for me to write it all out, so I’ll just link to it in the sources.
But I will write out a shorter solution that isn’t really a solution since it doesn’t cover all the possible cases, but it’s good enough for the purposes of the answer.
Here it goes:
Proof:
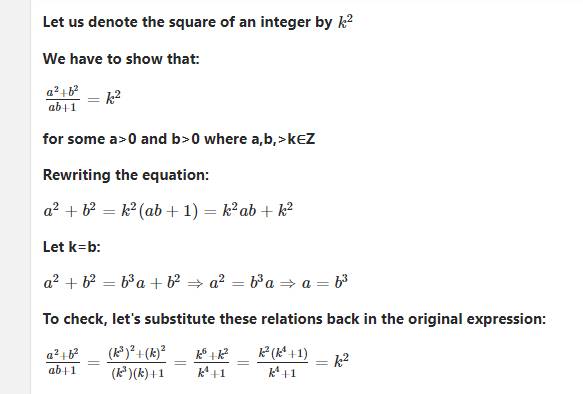
Yeah, by assuming k=b, we write off a bunch of possible cases, but hell, have you tried solving this? Sometimes average will have to do.
Conclusion
The runner up is the Twin Prime conjecture, that I worked on during my senior year in high school and first part of college. This conjecture had been unsolved for about 150 years before I convinced myself I solved it. (My number theory professor identified an ever so slightly small gap in my proof.)
In a several-way tie for first, I worked for a long time on trisecting an angle in 9th grade. Obviously, I succeeded. My geometry teacher could not find a problem in my construction, so it must have been a valid solution. Right…?
Oh, easily the Twin Prime Conjecture. I devoted far too much of my high school free time to looking into this problem, and managed to convince myself at least four different times that I finally had it.
You can guess pretty easily that I did not have it any of those four times. The fourth was actually the most rigorous to date, and my LATEX����� mock-up actually looked decently paper-like. I had managed to apply some sieve theory to get a direct formula for the twin-prime counting function.
The trouble was, when I discussed the finding with a professor, he noted that while my formula was “most likely correct”, it was pretty useless as well. The formula utilized probably the weakest sieve out there, and so trying to bound error terms and prove growth rates would be most probably harder to do than solve the conjecture by the actually foremost methods in the field.
And so, my high school optimism that I had the inklings of the next Terry Tao were reasonably squandered, and now at university I’m ready to really prepare myself to solve these great problems. With time, of course.
For me, it has been algebra. I understand the general theory of putting letters in math, but it confuses me quickly. X+3=9; solving for X isn’t hard; more than this will take me long enough to solve; I feel dumb. Then I see my sir making calculus to solve rocket science for some story or game. Sir says applied calculus is the hardest, but I have not studied calculus. There is also something about vectors.
The hardest type of math is probably just math you don’t understand.
Which is the hardest mathematical question in the world? math problem?